That Fictitious Force

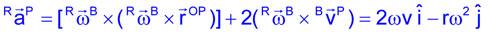
Imagine you are on a merry-go-round turning at a constant rotational speed. You are standing near its center, and you start walking at a constant speed along a straight line painted radially on its floor from its center to its outer edge. What forces will you feel? The answer to this question will help explain how a microelectromechanical system (MEMS) gyroscope works.

The gyroscope, invented in 1817, is used in vehicle control, aviation, aerospace, navigation, robotic, and military applications. MEMS gyroscopes have enabled applications in consumer electronics, such as camera stabilization and interactive video games. They are also used in numerous smartphone apps.

Engineers know that the forces of significance in everyday practice are physical contact forces (like friction) and forces that act at a distance (i.e., electromagnetic and gravitational). But what about the inertia force, the centrifugal force, and the mysterious Coriolis force, named for Gaspard Gustave Coriolis (1792-1843)? Aren't they real forces? No.

The inertia force (mass times absolute acceleration) is equal to the physical force you must apply to a mass to cause it to accelerate. The centrifugal force (mv²/r) is equal to the physical force you need to apply perpendicular to the trajectory of the mass to cause it to move along a circular path of radius r at a constant speed. We have all experienced these. But what is the Coriolis force? Let's answer the question posed at the start.

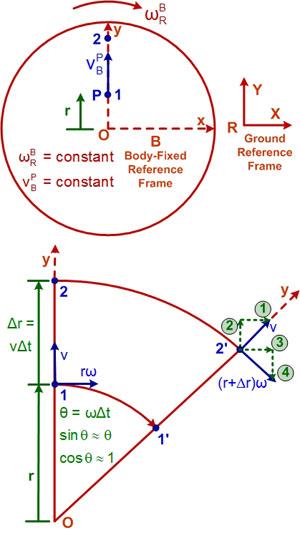
If we know our absolute acceleration during this motion, i.e., with respect to the ground reference frame R, then we know the forces we will feel. The absolute acceleration is the difference between the absolute velocity at points 2' and 1 divided by ∆t, in the limit as ∆t -> 0. First consider the Y direction acceleration: ∆v_Y = [component 2 + component 4] - v. Divide this by ∆t, and take the limit as ∆t -> 0. The result is that a_Y = -rω². This is called the centripetal acceleration and is due solely to component 4. The velocity v has no effect on the centripetal acceleration, and it depends only on position r and angular speed ω.

Next consider the X direction acceleration: ∆v_X = [component 1 + component 3] - rω. Divide this by ∆t, and take the limit as ∆t -> 0. The result is that a_X = 2ωv. This is called the Coriolis acceleration and is independent of position r. It represents the sum of two identical contributions: The effect of ω changing the orientation of v is exactly the same as the effect of v carrying rω to a different radius, changing its magnitude. In vector notation, the expression for the absolute acceleration of point P, in terms of unit vectors of the body-fixed axes, is

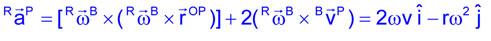
A gyroscope is a device that measures the angular velocity ω of a body about a certain axis of rotation. Classical gyroscopes are bulky, expensive, and unreliable. MEMS technology has the advantages of batch fabrication, small size, and low price. Almost all MEMS gyroscopes rely on vibrating mechanical elements that are driven to oscillate in the plane of the chip and to respond to rotation by another vibratory motion in the same plane. The main principle of MEMS gyroscopes is the transfer of energy between two modes of vibration (the drive and the sense modes) through the Coriolis acceleration. A fundamental understanding of the Coriolis force led to the development of this exciting technology.

Vist the Mechatronics Zone on DesignNews.com for all the latest Mechatronics news.