'Control, Control, You Must Learn Control'
April 18, 2014
Twenty-plus years ago, while teaching at Rensselaer Polytechnic Institute, my colleagues and I, working in the emerging field called "mechatronics," came to understand the importance of integrating control into the design process from the very start.
Adopting a model-based design approach made this integration easy indeed, accessible to all engineers with some education in control fundamentals and their application to real engineering systems. The increase in the power of microelectronics and the decrease in their cost has now made possible, in everyday systems, the application of control approaches found only in research labs a few years ago. Yes, now control is an indispensable technology in modern multidisciplinary engineering practice.
Alas, you would not come to that conclusion reviewing the engineering curricula at universities or speaking with most practicing engineers today who are products of those programs. Modeling and control make physics and mathematics relevant and necessary for the engineering student, and this message needs to be delivered on day one. Why is this not so? If you have an answer, please let me know.
Classical Control Theory (root-locus and frequency response analysis and design, i.e., transform methods) is applicable to linear, time-invariant, single-input/single-output (SISO) systems. This is a complex frequency-domain approach. The transfer function relates the input to output and does not show internal system behavior.
Modern Control Theory (state-space analysis and design) is applicable to linear or nonlinear, time-varying or time-invariant, multiple-input/multiple-output (MIMO) systems. This is a time-domain approach. This state-space system description provides a complete internal description of the system, including the flow of internal energy.
The aim of both techniques is to find a controller that satisfies the design specifications. Knowledge of both approaches, modern and classical, is essential to produce the best designs.
Even when control is taught, the subject of state variables and state-space control design is rarely covered. While classical control, specifically proportional-integral (PI) control, accounts for about 90% of industry applications, that is rapidly changing. State-space control is now essential for many applications to just function.
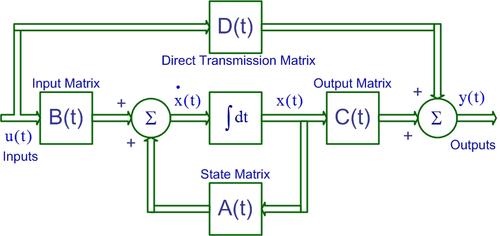
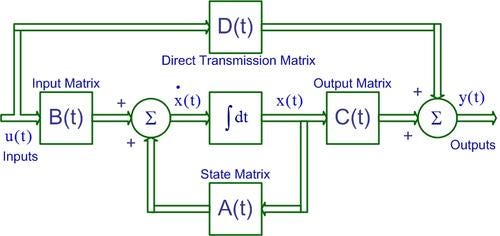
The state-variable equations (see diagram above) are a coupled set of first-order, ordinary, differential equations where the derivative of each state variable is expressed as an algebraic function of state variables, inputs, and possibly time. The state variables are independent variables capable of defining the state from which one can completely describe the system behavior. These variables completely describe the effect of the past history of the system on its response in the future.
Choice of state variables is not unique, and they are often, but not necessarily, physical variables of the system. They are usually related to the energy stored in each of the system's energy-storing elements, since any energy initially stored in these elements can affect the response of the system at a later time. The internal energy can always be computed from the state variables.
The number of elements in the state vector is unique, and is known as the order of the system. Advantages of state-space design are especially apparent when the system to be controlled has more than one control input or more than one sensed output, the MIMO system case.
Yoda was right, and his words need to be heeded today more than ever before.
Related posts:
About the Author(s)
You May Also Like



