Edgard Ngaboyamahina Innovation Advisor at RTI International
Sustainability
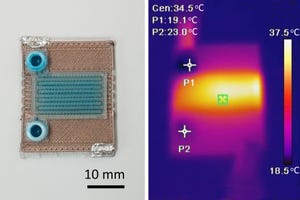
Ensuring Today’s Innovations Do Not Become Tomorrow’s ProblemsEnsuring Today’s Innovations Do Not Become Tomorrow’s Problems
Consider these tips for engineering sustainability into products and processes without compromising performance and safety.
Sign up for the Design News Daily newsletter.









.jpg_(1).png?width=300&auto=webp&quality=80&disable=upscale)